Department of Economics
ECON 4001.02
Fall 2023
Problem Set #3
Due by Wednesday, November 15th 2023 at 12:45 PM
NOTE: Please email your solutions to Wonjun Lyou ([email protected]) or turn them in at the start of class.
Problem 1 (14.2)
Suppose a monopolist faces demand q = 70 − p (or, equivalently, p = 70 − q).
Problem 1.1 Suppose marginal cost and average cost are constant at 6. What output level will maximize profits for the monopolist? What are the associated price and profit levels?
Problem 1.2 Assume now costs are c(q) = 0.25q 2 − 5q + 300. With the same demand curve, what will be the profit-maximizing quantity? What price and profit result?
Problem 1.3 Now use the cost function c(q) =1/75q3 − 5q + 250 and calculate the optimal quantity and resulting price and profit. Hint: set MC = MR as usual and use the quadratic formula to solve for q. You may want to use a computer to help.
Problem 1.4 Graph the demand curve and MR curve. Also graph the three MC curves from the above 3 problems, and identify how the optimal quantity changes as the cost function changes. You can use Desmos or another graphing program to do this.
Problem 2 (14.6)
A monopolist produces a good at a constant marginal cost of 5 dollars. It sells that good in two separate cities, labeled 1 and 2. Their demand curve for city 1 is q1 = 55 − p1 (or, equivalently, p1 = 55 − q1). The demand curve for city 2 is q2 = 70 − 2p2 (or, equivalently, p2 = 35 − 1/2 q2).
First suppose it’s impossible to travel between cities (for example, gas is extremely expensive). The monopolist therefore treats both markets separately, choosing p1 in city 1 and p2 in city 2.
Problem 2.1 What is the optimal quantity produced in each city? What are the resulting prices and profits in each city, and total profits overall? (Hint: you should find that p∗1 = p∗2 + 10.)
Now suppose that buyers in one city could go to the other market and buy their goods there if they wish, at a cost of $5 in gas. (If a consumer travels to another city then they pay $5, but the monopolist doesn’t get that $5.) But the quantity they buy is still determined by their original demand curve. So, for example, if all consumers purchase in city 2 at price p2 then the total quantity sold would be (55 − p2) + (70 − 2p2) (since consumers from city 1 would buy (55 − p2) units at price p2 and consumers from city 2 would buy (70 − 2p2) units at price p2).
Problem 2.2 If gas is $5, which city do customers go to if p1 + 5 < p2? Which do they go to if p1 > p2 + 5? And what happens if p1 − 5 ≤ p2 ≤ p1 + 5? What is the monopolist’s maximum profit in each of these scenarios? Which scenario is optimal for the monopolist? What will be their optimal prices p∗1 and p∗2 ? (Hint: from the previous problem, if consumers stay in their own cities then the monopolist would want to charge p1∗ = p∗2 + 10. But if that’s the case then people in city 1 would drive to city 2! So if the monopolist wants to keep consumers in their home city then it has to set p∗1 = p∗2 + 5.)
Finally, suppose that gas is free, so customers can travel between cities at no cost. They simply purchase from which ever city has the lowest price. Thus, the monopolist is forced to pick a single price p in both cities.
Problem 2.3 If gas is free, what will be the optimal price p ∗ , resulting quantities in each city, and total profit?
Problem 2.4 Comparing your answers to the previous three problems, how was the monopolist able to price discriminate when consumers can’t travel, or when travel was costly? In which situation did they make the most profit? Why?
Problem 3: Perfect Competition Becomes Monopoly
Suppose there are n ≥ 2 firms in a perfectly competitive market. There is only one good, so the partial equilibrium model applies. Each firm i chooses a quantity qi . They all have the same cost function: C(qi) = aqi + b/2qi2 + 2b, where a and b are positive numbers. (Notice the fixed cost of 2b.) The market demand is given by D(p) = d − ep, where d and e are positive numbers. We assume d − e(a + 2b) ≥ 4 and d − e(a + 2b) ≥ 2a(1 − e).
These problems require a lot of algebraic manipulation. If you really get stuck, you may use the following specific values: a = 2, b = 2, e = 1, and d = 106. Also, the answers to each problems are given at the end of this problem set. If you get stuck on an early problem, you can just use the correct answer to proceed to the next problem. Of course, to get credit you must show your work! Simply copying the answers will not earn you any points.
Problem 3.1 What will be the short-run supply function for each firm? Don’t forget about the shut-down point.
Problem 3.2 Solve for the price p¯ below which the firm shuts down in the short run. (You can denote the associated shut-down quantity by q¯.)
Problem 3.3 What will be the entire market’s short-run supply function? Let Qc denote the total quantity supplied in this perfectly competitive market. (Hint: fix a price p ≥ p¯ and then figure out Qc for that price. And remember if each firm supplies q then the total supply is simply nq since there are n firms.)
Problem 3.4 What would be the (short-run) competitive equilibrium price (where supply equals demand)? Denote it by p∗c .
Problem 3.5 What quantity does each firm i produce in competitive equilibrium? Denote this by qi∗.
Problem 3.6 Let p0 be the price at which firms make zero profit, and let q0 be the quantity they produce at price p0. We know that if p ∗c > p0 then p ∗ c = MC(qi ∗ ) > AC(qi ∗ ). This means firms are making economic profits. But then new firms will enter the market, causing n to increase. As a result, p ∗ c and qi ∗ will both decrease, causing equilibrium profits to decrease. The zero-profit condition is given by MC(q0) = AC(q0). Find q0 and then find the long-run number of firms such that profits are exactly zero. Denote that by n ∗ . (Hint: all firms will be supplying exactly their zero-profit quantity q0 and the market price will be p0. Since total supply equals total demand in equilibrium, that means q0n ∗ = D(p0). But also we know p0 = MC(q0). Plugging that in to the previous equation gives q0n ∗ = D(MC(q0)). Once you know q0 you can use this equation to find n ∗ .)
Problem 3.7 Calculate Qc and p ∗ c when n = n ∗ . This is the long-run equilibrium. (Hint: qi = q0, Qc = n ∗ qi , and p ∗ c = MC(qi).) Now suppose all n ∗ firms merge into one large monopoly. It chooses quantity qm and sells at the price pm such that D(pm) = qm. Its cost function is C(qm) = aqm + b/2n∗ q2m + 2b. Notice here that n ∗ is just a parameter (a single number); it’s no longer representing the number of firms, since there is only 1 firm.
Problem 3.8 Given the market demand function D(p), solve for the ‘inverse demand function’. That is, for any quantity qm, find the price P(qm) that the monopolist can charge.
Problem 3.9 Verify that the monopolist’s marginal cost curve exactly matches the market supply that existed before the merger (at least for qm ≥ q¯).
Problem 3.10 Solve for the monopolist’s profit-maximizing quantity q∗m. (You can leave it as a function of n ∗ .)
Problem 3.11 Remember that, before the merger, the n ∗ firms were producing a total output of Qc = n ∗ q0. Verify that the total output under the monopolist is smaller (q∗m < n∗ q0). (Hint: plug in your solution for n ∗ , and remember d − e(a + 2b) is positive.)
Problem 3.12 Solve for the monopolist’s price P(q∗m) and compare it to the perfectly competitive equilibrium price p ∗c .
Problem 4: Differentiated Products with n Firms
Suppose there are n ≥ 2 firms whose goods are substitutes. Each firm chooses its price pi . Let P = p1 + p2 + · · · + pn be the sum of all prices, and P−i = P − pi be the sum of all prices except firm i. Each firm i’s demand is then given by qi = 120/n − pi + P−i/2(n −1) . In other words, if the average price of other firms (which is P−i/(n−1)) goes up, then firm i’s demand goes up. Also, the number of consumers who would buy from firm i if all prices were zero is 120/n, which decreases as n increases. Firms have a constant marginal cost, which we’ll assume is zero for simplicity.
Problem 4.1 Suppose all firms set the competitive price, which is zero since marginal costs are zero. How many units would be sold to each firm i?
Problem 4.2 At the competitive price (pi = 0 for all firms), how does the total quantity sold (nqi) change as n → ∞?
Problem 4.3 Solve for firm i’s best response function BRi(P−i).
Problem 4.4 There is a Nash equilibrium of this game in which all firms choose the same price p ∗ . Find p ∗ . (Hint: in equilibrium each firm is best responding to P∗−i = (n − 1)p ∗ .)
Problem 4.5 What is each firm’s quantity sold in equilibrium (qi ∗ )?
Problem 4.6 What is each firm’s profit in equilibrium (πi ∗ )?
Problem 4.7 How do the equilibrium price p ∗ , quantity qi ∗ , and profit πi ∗ change as n → ∞? (Remember, the perfectly competitive price would be zero since marginal costs are zero.)
Problem 4.8 How do total industry profits (given by nπi ∗ ) change as n → ∞?
Problem 5 (based on 8.4 in the book)
Two neighbors (labeled i = 1 and i = 2) simultaneously choose how many hours to spend main-taining their lawn. Let hi be the hours chosen by player i. The total payoff to the two players are
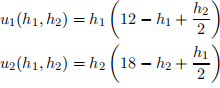
Problem 5.1 Calculate the best response functions BR1(h2) and BR2(h1).
Problem 5.2 Suppose one neighbor decides to spend one more hour maintaining their lawn. Will the other neighbor respond by spending more or less time on their own lawn. How many minutes more or less?
Problem 5.3 Calculate the Nash equilibrium hours for each player. Recall that the Nash equilib-rium can be solved from the formula h*1 = BR1(BR2(h∗1)).