Hello, if you have any need, please feel free to consult us, this is my wechat: wx91due
EE3025 - Statistical Methods in ECE
Spring 2024
Homework 1
Due on Thursday, Feb. 1, 2024, 5:00 PM
Problem 1
Let A, B, C be three sets as shown below.
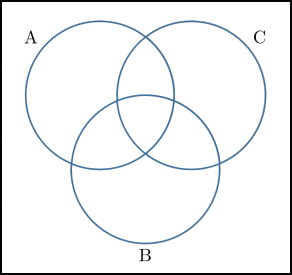
For each of the following sets, draw a Venn diagram and shade the area represented by the set.
(a) (A ∪ Bc ) ∩ (B ∪ Cc )
(b) A \ (B ∩ C)
(c) (A \ B) ∪ (B \ A)
(d) A ∪ (B ∩ C)
(e) (A ∪ B) ∩ C
(f) (A ∪ B) ∪ C
(g) (A − B) ∩ Cc
(h) A ∩ (B \ C)c
(i) B ∪ (Bc \ A)
(j) A \ (B \ C)
Problem 2
Let A = {1, 2, 3, ..., 10}, B = {−5, −3, −1, 1, 3, 5}, C = {2, 3, 5, 7, 11, 13, 17}.
(a) If S = {−6, −5, ..., 18, 19, 20}, draw a Venn diagram and specifiy the numbers belonging to each area.
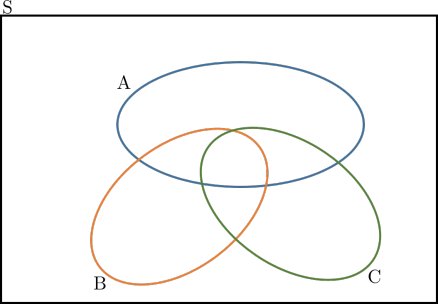
(b) Use this diagram to find the elements in
(i) (B ∪ Cc ) − (A ∪ Bc )
(ii) (C − A) ∪ (Bc ∩ A)
(iii) (A \ B) ∪ (B \ A)
(iv) C ∩ (Ac − Bc )
Problem 3
We have two dice of colors red and blue. Each die has 6 sides each with a different number from {1, 2, 3, 4, 5, 6}. We roll the dice and observe the upward facing numbers. Each outcome has the form (r, b). The sample space is {(1, 1), (1, 2), (1, 3), . . . , (6, 6)}. Write the set representing each event below.
(a) The outcome of the red die is an odd number and the blue die shows a prime number.
(b) The number on the red die is larger than the number on the blue die.
(c) The sum of the red and blue dice is 8.
(d) The product of the red and blue dice is 6.
(e) The numbers on both dice are the same.
(f) The number on the red die minus the number on the blue die is at least 2.
(g) The sum of the red and blue dice is at most 5.
Problem 4
Consider a random experiment with S = {x,y,z, t}. Let P({x,y, t}) = 0.8 and P({y, z}) = 0.6. First show that it is a valid probability space. Then, use the Axioms of Probability and properties of Set Theory to compute the following probabilities (find the minimum and maximum values if the exact probability cannot be uniquely determined).
(a) P({x,t})
(b) P({y})
(c) P({z})
(d) P({x})
(e) P({x,y,z, t})
(f) P({t,y})
Problem 5
Let A ⊆ B and A ∩ C = 。. Use the Venn Diagram to determine whether each statement below is true or false. If it is true, then analytically prove it (using properties). If it is false, determine under what additional conditions on A, B, and C it can be true.
(a) (A ∪ (B ∩ C)) − B = 。
(b) (C ∪ B) − (B − A) ⊆ C ∪ (A ∩ B)
(c) (B − C) ∩ (B − A) = B − (A ∩ C)
(d) A ⊆ (A ∩ (B − C)) ∪ (A − (B ∩ C))