Hello, if you have any need, please feel free to consult us, this is my wechat: wx91due

MATH21112 Rings and Fields Example Sheet 6

More on Homomorphisms and Ideals

1.  Show that the map from Z[√2] to itself given by sending a + b√2 to a -b√2 is an automorphism.  Show that there are no other automorphisms of Z[√2] apart from the identity map.

2.  Let  R = Z [√2] and let 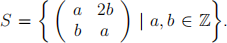 Define the map  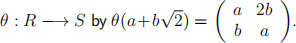Prove that θ is an isomorphism. (You may assume that S is a ring.)

3.  Prove that  if θ : R -→ S is a surjective  homomorphism of rings and R is commutative then S must be commutative.  Give an example to show that the conclusion may be false if θ is not surjective.

4.  Suppose that R is a commutative ring of characteristic 3.  Prove that the map θ  : R  -→  R defined  by  θ(r) = r3   is a  homomorphism.   What  is ker(θ)?

5.  Give an example of a ring R and a subset H of R which contains 0 and is closed under addition and multiplication but which is not an ideal of R.

6.  Prove that  if θ : R -→ S is a surjective homomorphism of rings and I is an ideal of R then θ(I) is an ideal of S.  Give an example to show that the conclusion may be false if θ is not surjective.