Hello, if you have any need, please feel free to consult us, this is my wechat: wx91due
ECON0013
PART A
You must answer the question in this section.
A.1 (a) Fred consumes two goods, apples q1 and pears q2 , purchased at prices p1 and p2 from a budget y. His preferences are described by utility function
u(q1 , q2 ) = q1 + ln (q2 + 1) .
Assume that y > p1 - p2 > 0.
(i) Show that his Marshallian demands are
q1 = f1 (y, p1 , p2 ) = (y + p2) /p1 - 1
q2 = f2 (y, p1 , p2 ) = p1 /p2 - 1.
(ii) Find his expenditure function c (o, p1 , p2 ) (where o denotes utility) and use it to show that his Hicksian demands are
q1 = g1 (o, p1 , p2 ) = o - ln (p1 /p2)
q2 = g2 (o, p1 , p2 ) = p1 /p2 - 1.
(iii) Let the following define elasticities relating to the Marshallian and Hicksian demands:
η*11 = ∂ lng1 /∂ ln p1
η 11 = ∂ ln f1 /∂ ln p1
E1 = ∂ ln f1 /∂ lny
and let σ1 = p1 q1 /y denote the budget share of apples. Find expressions for these elasticities, show that
η 11 = η*11 - σ 1E1
and ofer an interpretation.
(iv) Suppose that p1 doubles while p2 is unchanged. Define the true cost-of-living index
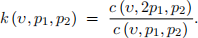
Is k (o, p1 , p2 ) increasing or decreasing in o? Why is that and what does this tell us?
(b) Freda owns a farm which produces apples Q using two inputs, skilled labour z1 and unskilled labour z2 , purchased at input prices w1 and w2. The firm’s production possibilities are described by production function
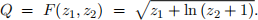
(i) Without detailing the solution to any further minimisation exercise, use the results de-rived above to establish the form of her conditional input demands, h1 (Q, w1 , w2) and h2 (Q, w1 , w2 ), and her cost function C (Q, w1 , w2 ). Assume that w1 > w2 .
(ii) Suppose that Freda is contracted to an employer to produce apples. She is given a fixed budget Y to purchase labour inputs and instructed to produce the greatest number of apples that she can without going over that budget. Assume that Y > w1 - w2 . By relating her decision problem to those of earlier parts, comment on what the earlier derived expression
η 11 = η*11 - σ 1E1
tells you about how her input decisions respond to changes in input prices.
(iii) Suppose that Freda decides to leave this employment contract and sell her firm’s apples herself. She takes the price of apples P as given and chooses output to maximise profit
π(Q) = PQ - C (Q, w1 , w2).
Find her unconditional input demands d1 (P, w1 , w2) and d2 (P, w1 , w2) and her output supply function s (P, w1 , w2).
(iv) Let the following define elasticities relating to the conditional and unconditional input demand and output supply functions:
H*11 = ∂ lnh1 /∂ ln w1
H11 = ∂ lnd1 /∂ ln w1
Σ 1 = ∂ ln s/∂ ln w1
E1 = ∂ lnh1 /∂ ln Q.
Is there a link between H*11 , H11 , Σ 1 and E1 applicable to this scenario? If so, present it, calculate the terms in the formula and interpret it.
(v) Suppose that w1 doubles while w2 is unchanged. How would you interpret the ratio
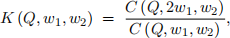
how does this vary with Q and what does this tell us?
PART B.I
Answer ONE question from this section.
B.I.1 There are two firms: Firm A and Firm B. Firm A is a leader and uses two inputs, labour xh and capital xk, to produce its output zA . The markets for labour and capital are perfectly competitive and w is the price of labour and r is the price of capital. Firm A has the production function zA = √xkxh . Firm A is a monopoly supplier of the good zA and it sets the price p for its output. Firm B, the follower, uses two inputs, labour zh and the output zA of Firm A to produce its output y. It has the production function y = (zA zh)1/3 and can sell its output on the world market at the price m.
(a) What are the input demands and profits of Firm B?
(b) What is the cost function of Firm A? What is Firm A’s optimal price? What is Firm A’s profit?
(c) Are there other assumptions about how the price that firm A’s product gets determined that we could make? How would these assumptions alter economic outcome of this game?
(d) Firm A and B merge into a single profit-maximizing firm that is called Firm AB. Describe Firm AB’s behaviour and compare it with what you found above.
(e) Would a regulator be happy that this merger had taken place?
B.I.2 A worker is employed by a firm to produce output. If the worker puts in efort there is: probability p that they produce two units of output, probability q that they produce one unit, and probability 1 - p - q that they produce zero units. If the worker does not put in efort these probabilities are: r , s, 1 - r - s respectively. The manager decides to pay the worker u ≥ 0 if two units are produced v ≥ 0 if only one unit is produced and w ≥ 0 if no units are produced. The worker has a utility function b(x) - c ifshe receives the wage x = w, v, u and puts in efort. If she does not put in efort she has the utility b(x). The worker can earn the utility U from working elsewhere. The manager can sell each unit of the good that the worker produces for a price R.
(a) What conditions must the wage contract (u,v, w) satisfy if the worker is willing to work at the firm?
(b) What is the worker’s expected marginal revenue product for efort?
(c) What conditions must the wage contract (u,v, w) satisfy if the worker prefers low to high efort?
(d) The firm decides that it is content with low efort from the worker and b(x) = √x. Write down and solve a constrained optimisation that describes the cheapest way for the firm to achieve this. Interpret what you find. When does the firm make a profit?
(e) The firm decides that it is content with low efort from the worker and b(x) = x2 . Write down and solve a constrained optimisation that describes the cheapest way for the firm to achieve this. Interpret what you find. When does the firm make a profit?
(f) Assume that p ≥ r and q ≥ s. The firm decides it wants high efort from the worker and b(x) = √x. But the firm ignores the possibility that the worker might leave and go elsewhere. Write down the constrained optimisation that describes the firm’s cost minimisation in this case. Explain why w = 0 at an optimal solution to this problem. Hence describe the complete cost minimising solution in this case.
(g) Does the answer you have found in the previous question tell us much about the optimal contract the firm will ofer the worker when b(x) = √x? What do you expect this optimal contract will look like?
PART B.II
Answer ONE question from this section.
B.II.1 (a) A consumer is observed in two periods t = A, B. Two goods are consumed, food q1(t) and cloth q2(t). Prices are p1(t) and p2(t). Budget shares are
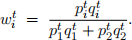
Laspeyres and Paasche cost of living indices, L and P , are defined as ratios of costs of purchasing initial and final bundles
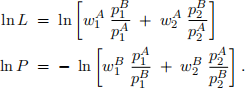
The Fisher index F is the geometric mean of the Laspeyres and Paasche indices
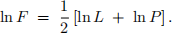
The To… rnqvist index T is the average-budget-share-weighted geometric mean of the price ratios
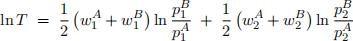
(i) Explain what is meant in this context by a true or Kon u…s cost of living index.
(ii) Suppose the consumer has preferences defined by utility function
u (q1 , q2 ) = min [aq1 , (1 - a)q2]
for preference parameter 0 < a < 1. Which if any of the four indices, L, P , F and T , coincides with a true cost of living index? Comment.
(iii) Suppose the consumer has preferences defined by utility function
u (q1 , q2 ) = Q ln q1 + (1 - Q) ln q2
for preference parameter 0 < Q < 1. Which if any of the four indices, L, P , F and T , coincides with a true cost of living index? Comment.
(b) The economy of Ricardia is based on consumption of food and cloth. Laspeyres, Paasche, Fisher and To… rnqvist indices are calculated using prices and aggregate quantity data for periods t = 1 to 25.
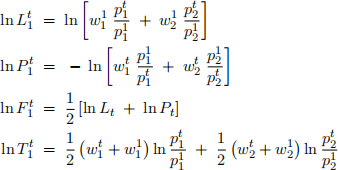
These four indices are shown in Figure 1.
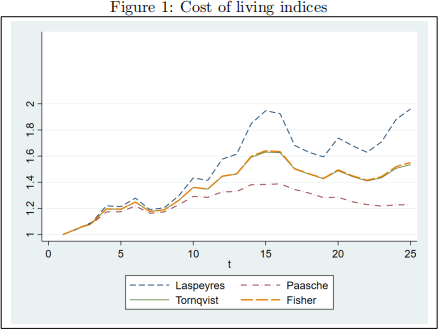
(i) Comment on the fact that Lt > Tt > Pt for all t > 1.
(ii) The treasury of Ricardia wants to use one of these indices to uprate state benefits. What would you advise?