Hello, if you have any need, please feel free to consult us, this is my wechat: wx91due
MATH 351, SPING 2024
Assignment #2
Due: June 5.
1. (On equivalence of norms) Let || · || and ||| · ||| be two norms defined on a R-vector space V . We let
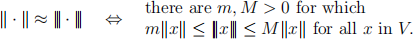
(a) Verify that ≈ is an equivalence relation on the set N(V ) of norms on V .
(b) Consider the vector space R n . Show that for 1 < p < r < ∞ that
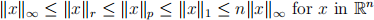
and deduce that these norms are all equivalent.
[Hint: for the hardest of these inequlities, if x ≠ 0, first divide ||x||rr by ||x||∞r, notice that t r ≤ t p if 0 ≤ t ≤ 1.]
(c) Show that for x in R n we have ||x||∞ = limp→∞ ||x||p.
(d) Show that for 1 ≤ p < r < ∞ we have a proper containment relations
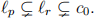
[The integral test for series convergence is your friend.]
(e) Show that for 1 ≤ p < r ≤ ∞, || · ||p 6≈ ||· ||r on ` 1. (Recall, from above, that ` 1 ⊆ ` p for each 1 ≤ p ≤ ∞.)
2. (On equivalence of metrics)
Let X be a non-empty set and M(X) ⊂ [0,∞) X×X denote the set of all metrics on X. For d, ρ in M(X) let
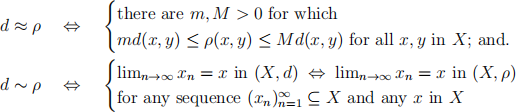
(a) Verify that ≈ and ∼ are equivalence relations on M(X).
(b) Show that d ≈ ρ in M(X) implies that d ∼ ρ
(c) Show that d ∼ ρ in M(X) ⇔ (X, d) and (X, ρ) admit the same open sets.
(d) Let d ∈ M(X) and f : [0,∞) → [0,∞) satisfy that
f(0) = 0 and f is strictly increasing, subadditive and continuous. (♥)
Show that df : X × X → [0, ∞), df (x, y) = f(d(x, y)) defines a metric with df ∼ d. Furthermore, show that f(s) = s+1/s satisfies (♥).
(e) Does d ∼ ρ in M(X) imply that d ≈ ρ? Prove this, or supply a counterexample.
3. We say that a metric space (X, d) is separable if there is a countable set Z = {zk} ∞k=1 ⊆ X with closure Z = X.
(a) Show that if (X, d) is separable, then it cardinality satisfies |X| ≤ c.
[Hint: this has aspects similar to our construction of R from Q.]
(b) Show that for 1 ≤ p < ∞ that ` p is separable.
(c) Let C(R) = {f ∈ R R : f is continuous}. Show that |C(R)| = c.
[Hint: a continuous function is determined by its behaviour on Q.]
(d) Show that ` ∞ is not separable.
[Hint: first find a subset X of elements which is uncountable and for which k χ − χ 0 k ∞ ≥ 1 for χ = χ 0 in X.]
(e) Show that |` ∞| = c.
4. In R with usual metric d(x, y) = |x − y| let
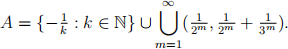
(a) Compute the interior A◦ , boundary ∂A, derived set A0 , and closure A, in R.
(b) Let now B = {− k/1 : k ∈ N}. Compute the interior, boundary, derived set, and closure but relativized in A. [You might wish to write, B◦A, ∂AB, B'A, BA for these relativized sets.]