Hello, if you have any need, please feel free to consult us, this is my wechat: wx91due
EEEN3005J: Communication Theory
SEMESTER II FINAL EXAMINATION - 2018/2019
Question 1:
A baseband signal, g (t) , with bandwidth 10 kHz, is such that its amplitude satisfies jg (t)j < 0.8 V, and has average power = 0.1 Watts. This signal is connected to a circuit composed of a mixer and an adder as shown in Figure 1 below. The resulting signal, ˜(s)(t) , is transmitted through a channel as shown. The scaling factor, α , represents a signal power attenuation of 120 dB, and w (t) is additive white Gaussian noise with single-sided power spectral density (PSD) N0 = -174 dBm/Hz. The receiver applies an ideal band pass filter having bandwidth just su伍cient to capture the whole of the received modulated signal.
[Note that if the power of a signal is Pm mWatts, then its power in dBm is = 10 log10(Pm ) dBm.]
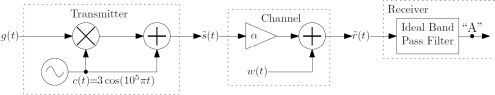
Figure 1: Circuit to be considered in question 1
1. Sketch the time-domain signal, ˜(s)(t), showing numbers and units on both axes. What name is given to this type of modulation?
2. Calculate the signal-to-noise ratio (SNR) in dB at point “A” . Do not count the carrier component as contributing to the “signal power” here, as it bears no information.
3. What do we mean when we say that a random signal is wide-sense stationary?
4. What is meant by the Energy Spectral Density (ESD) of a deterministic energy sig- nal?Consider a signal,x (t), with one-sided ESD given by:
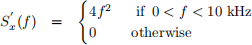
Determine the fraction of the signal’s energy that lies in the frequency range 0 < f < 5 kHz
Question 2:
Consider the circuit in Figure 2 where the control signal c (t), that is guaranteed to be either ±1 V, is used to control the position of the two switches as shown.
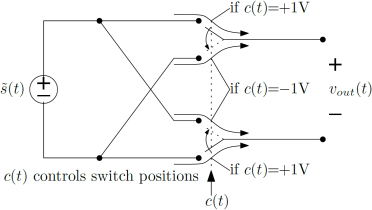
Figure 2: Circuit to be considered in question 2
1. Write a simple expression for the circuit’s output, vout (t), in terms of c (t) and ˜(s)(t), explaining your answer by clearly describing the operation of the circuit when c (t) = +1 V and when c (t) = -1 V.
2. Let ˜(s)(t) be a bandpass signal with centre frequency fc Hz, and c (t) be a square wave signal alternating between ±1 V also with frequency fc Hz. In this case
• sketch a possible power spectral density (PSD) for ˜s(t)
• sketch the PSD of c (t)
• sketch the resulting PSD of vout (t)
3. Provide a clear definition of \Double SideBand Suppressed Carrier (DSB-SC)" modu- lation. Your answer should contain the mathematical expressions and derivations of a DSB-SC signal in the time and frequency domain. You should also include sketches of an example DSB-SC signal in both domains.
4. Explain how the circuit in Figure 2 could be used, in conjunction with some additional circuit elements, to demodulate a DSB-SC signal.
5. The process of demodulating a DSB-SC signal often sufers from local oscillator \phase and/or frequency mismatch" errors. Explain the meaning of this statement
Question 3:
1. What is the instantaneous frequency fi (t) of an arbitrary signal A (t) cos (φ (t))?
2. If a modulated signal can be written as Ac cos (2πfct + θ (t)) give an expression for the instantaneous frequency.
3. What is Frequency Modulation (FM)? your answer should derive an expression for ˜s(t), the FM signal, in terms of g (t) the modulating signal, and kf the frequency sensitivity.
4. Let g (t) be a sine wave with amplitude Am and frequency fm kHz.
• Derive an expression for ˜s (t) in terms of Am and fm.
• What is the modulation index β and modify the above expression to include β.
• Derive an expression for whats called ”the peak frequency deviation” ∆f . Hint: ∆f is should really be called the ”peak instantaneous frequency deviation”
• Why is the spectrum of this sinusoidal modulation FM signal, ˜s (t) a line spectra?
• The Fourier series for ˜s (t) is:
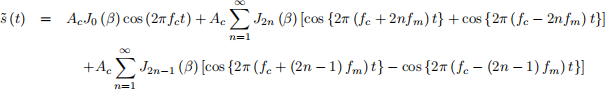
where Jn (β) is the nth order Bessel function of the first kind.
Let fm = 8kHz, and fc = 100MHz:
– Using Figure 3 sketch the spectra for β = 0.1
– If Am = 1Volt, what approximate value of frequency sensitivity kf would result in the spectral component at fc being zero? You answer should use the correct units for kf.
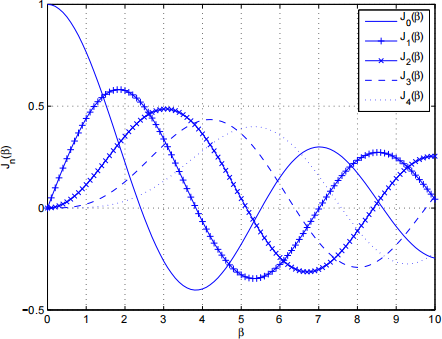
Figure 3: Some Bessel functions for use in question 3.
Question 4:
Consider the system shown in Figure 4.
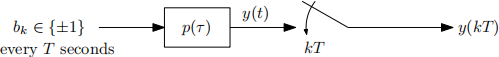
Figure 4: System to be considered in question 4
1. Write an expression for y (t).
2. Hence, or otherwise, derive (don’tjust quote) a time domain criteria on p (τ ) such that the collection of samples {y (kT)} are all ISI-free.
3. Derive (don’tjust quote) the frequency domain version of the criteria derived in part 2. Let T = 1 and P (f), as shown in Figure 5(a), be the Fourier transform of p (τ):
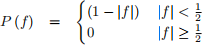
i.e. a triangular function of f.
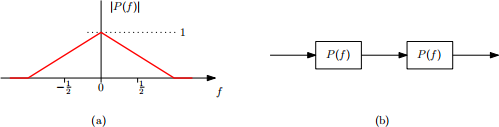
Figure 5: (a) Amplitude spectrum, jP (f)j, of pulse p (τ ) and (b) the cascade of two pulse shaping filters.
4. Is this p (τ ) an ISI-free pulse? Give reasons for your answer.
5. Is the cascade of two of these, as shown in Figure 5(b), ISI-free?
6. Suggest a simple modification to P (f) that would allow the cascade of two of them to be ISI-free.
7. Why is this an important result? Give an example of where it is commonly used.