Hello, if you have any need, please feel free to consult us, this is my wechat: wx91due
EE3025 - Statistical Methods in ECE
Spring 2024
Homework 4
Due Thursday, February 29, 2024, 9:00 PM
Problem 1
A factory manufactures and sells light bulbs in boxes, including an 8 bulb. The factory engineers believe that there is a p = 1/5 chance for each bulb to be defective, independent of all other lights.

(a) What is the probability mass function (PMF) of the number of defective bulbs in each box?
(b) What is the average number of defective bulbs in each box?
(c) A retailer who sells the bulb boxes, can return each box if it contains more than 2 defective bulbs. What fraction of bulb boxes will be returned to the factory?
(d) In order to improve its reputation, the factory hires a quality control technician to approve boxes. Jack opens each box, tests up to 3 lightbulbs from the box, and only approves a box to be sent to the retailer if none of the 3 tested bulbs are defective. Otherwise, he marks the box as “bad” . What is the chance for an approved box to be returned from the retailer?
(e) Assume Jack spends 25 seconds to test each lightbulb. What is the average time he spends on each box? (f) Let N be the number of defective lights in a “bad” box. What is the range (sample space) of N?
(g) Find PN (3), i.e., the probability that there are 3.
Hint: First, find the probability of N = 3 conditioned on the box is marked as bad on testing the first bulb.
Problem 2
Let X be a discrete random variable with p.m.f. given as
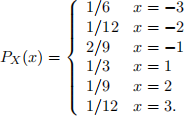
(a) Compute and draw the c.d.f. FX (x).
(b) Find E[X].
(c) Let Y = |X| . Find PY (y).
(d) Find E[Y] and compare it with |E[X]|.
(e) Compute PZ(z) for Z = 1/X.
(f) What is E[Z]? Is it equal for 1/E[X]?
(g) Compute E[X2].
Problem 3
The Binomial distribution, Bino(n,p), well approximates a Poisson distribution with a parameter λ = np, Pois(λ = np), when n is sufficiently large, but p is small so that np is not large, i.e. does not grow with n.
(a) Plot the probability mass functions (PMF) of Bino(n,p) and Pois(np) with the parameters n = 100, p = {0.2, 0.8} for the interval k = [0, 100]. You may want to use thematlab functions binopdf and poisspdf. What do you observe?
(b) Show that Pois(λ) approximates Bino(n,p) when n is large,p is small, and λ = np, i.e.,
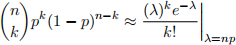
for small values of k.
Hint: First, note that fork relatively smaller than 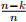 → 1 as n → ∞ . You might also need to use ln(1 −
→ 1 as n → ∞ . You might also need to use ln(1 −  )n ≈ −
)n ≈ −  ≈ 0.
≈ 0.

Death Star company starts to manufacture BB8 to celebrate new Star Wars movie. Suppose that 1 in 5000 BB8 is defective. Let X denote the number of
(c) defective BB8’s in a group of size 10000. What is a probability that at least 4 of them are defective? Find the exact probability and its approximation using the above result.
Problem 4
Let the CDF of a discrete RV X is
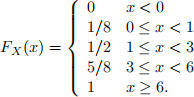
(a) What is the p.m.f. of the RV X?.
(b) Find the expected value of X, i.e. E[X]?
(c) Find the variance of the RV X?.
(d) Compute the variance of Z = X2.
(e) Let Y = 2X . Find E[Y].
Problem 5
We have an unfair coin with P(H) = 3/5 and P(T) = 2/5. We toss the coin until we observe 3 tails. Let X denote the number of coin tosses until we observe the 3rd tail.
(a) What if PX (7)?
(b) What type of random variable is X?
(c) What is the mode of X?
(d) Determine the medianofX.
(e) Compute the expected value of X.
Hint: You can use the identity
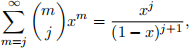
which holds for any x with |x| < 1.
(f) Find the variance of X.
Hint: You can use the identity n2 = n(n + 1) − n.