ECON 300 - Intermediate Microeconomics
Winter 2024
Problem Set 2
Due: January 29th, 2024
1. (30 points) Yang makes her own trail mix at home and likes to have a particular ratio of dried fruit (d) to peanuts (n). Her utility is represented by the following Leontief preferences over bags of dried fruit and peanuts:
u(d,n) = min(6d,18n)
The price of a bag of dried fruit is pd , the price of a bag of peanut is pn , and Yang’s income is represented by m.
(a) (2 points) Suppose Yang has 3 bags of peanuts (n), how many bags of dried fruit (d) must be consumed in order to have a utility 36?
(b) (6 points) Setup Yang’s utility maximization problem. Then find the Marshal- lian/Ordinary demand functions for goods d and n as functions of prices and income. Be sure to show all you work.
(c) (6 points; 2 points each) Calculate the price elasticity, cross-price elasticity, and income elasticity of Yang’s demand for dried fruit (d* ). Be sure to write out the elasticity formula for each and clearly show your calculations.
. Price elasticity of demand
. Cross-price elasticity of demand
. Income elasticity of demand
(d) (6 points) Note that all the exogeneous parameters (pd , pn , and m) are greater than 0. Using your calculations from part (c), explain whether Yang sees dried fruit as an ordinary, inelastic, or Giffen good? As a substitute or complement to peanuts? as a normal or inferior good?
(e) (6 points) Setup Yang’s expenditure minimization problem. Then find the Hick- sian/Compensated demand functions for goods d and n as functions of prices and U¯, the lowest acceptable level of utility. Be sure to show all your work.
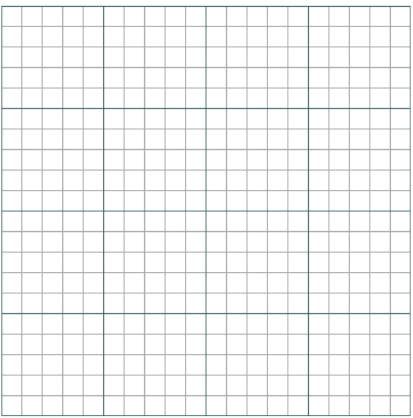
(f) (4 points) Let pn = 3, m = 20, and U(¯) = 72. Graph both Yang’s Marshal-
lian/Ordinary demand and Hicksian/Compensated demand for dried fruit (d) on the same grid below. Be sure to label the axes and the demand curves. Note that what you graph are actually inverse demand functions.
2. (40 points) Victoria has the following linear preferences over Brazilian chocolates (x) and French pastries (y):
u(x,y) = 35x + 28y
(a) (6 points) Solve for Victoria’s Marshallian demand for Brazilian chocolates and French pastries for prices and income px , py ,m.
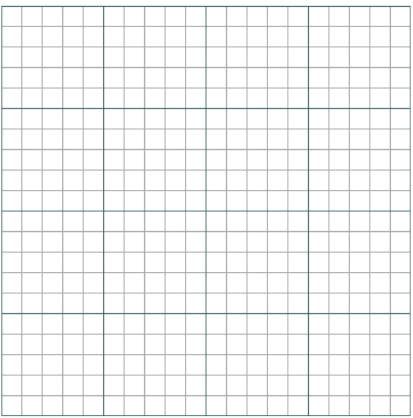
(b) (5 points) Graph Victoria’s Marshallian/Ordinary demand function for Brazilian chocolates x* (px ) when her income is m = 40 and the price of French pastries is py = 8. Make sure to show your calculations for how you identified the demand functions / key points you plotted.
(c) (6 points) Now suppose, in addition to py = 8 and m = 40 from (b), px = 7. Based on your answers in (a), determine Victoria’s demand for each good x* and y* . Show the marginal utilities per dollar as part of your answer. Your answer should fall in one of the three cases in (a). Besides, What is the maximized level of utility?
(d) (5 points) Graph Victoria’s indifference curves that represent utility of 50, 100, 150, and 200 on the graph below. You should be drawing 4 indifference curves, make sure to label with one represents which utility level. Make sure to also show your calculations for how you identified the form of the indifference curves.
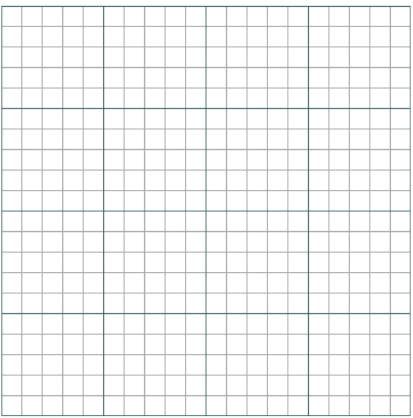
(e) (4 points) Recall that px = 7, py = 8, and m = 40. Graph Victoria’s budget constraint on the plot above. Explain how this graphically reinforces your answer from part (c). Show your work for calculating the slopes of the budget constraint and indifference curves.
(f) (6 points) Victoria is reconsidering her consumption strategy. Instead of utility maximizing, she wants to minimize the expenditure. Suppose French pastries still costs $8, but Brazilian chocolates price isn’t specified (leave aspx ). The minimum level of utility Victoria wants is U(¯) = 200. Setup and solve the expenditure minimization problem for xc (px ) and yc (px ).
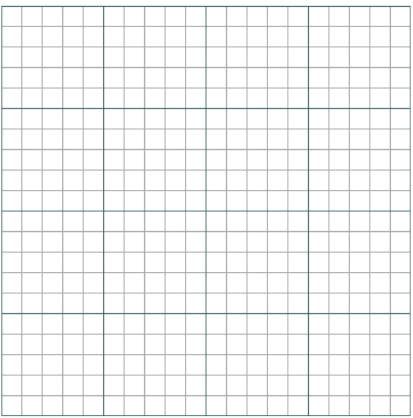
(g) (5 points) Graph the Hicksian/Compensated demand function for Brazilian choco- lates (xC (px )). Be sure to label the graph and show your work.
(h) (3 points) Under what condition the following two statements are both satisfied?
. The Hicksian demand in (f) is equal to the Marshallian demand you solved in (c) or (e), i.e., xc = x* .
. the minimized expenditure is equal to the income given in the utility maxi- mization problem, i.e., E(px , pg ,U(¯)) = m = 40.
Hint: Think about the value of px . Do not overthink and calculation is for veri- fication purpose only. p.s. You certainly don’t need all the empty spaces in this page (Ijust don’t want to start question 3 here) .
3. (35 points) Mark has preferences defined over Starbucks frappuccinos (good x) and
scones (good y). The preferences are represented by the following utility function:
u(x,y) = ln(4x7y2 )
The costs are px and py for frappuccino and scone, respectively. He has m dollars to spend.
Hint: You may find it helpful to rewrite the utility function for the utility maximization problem but use the original utility function for the expenditure minimization problem.
(a) (2 points) Set up Mark’s utility maximization problem.
(b) (4 points) Can you use the Lagrangian method to solve this optimization prob- lem? If yes, explain how you know and check the sufficient condition for interior solution. If not, explain how you know and describe what method is appropriate to solve this problem.
(c) (9 points) Solve Mark’s utility maximization problem for his Marshallian/Ordi- nary demand functions x* (px ,py , m) and y* (px ,py , m) using the method you de- cided was appropriate in the previous part. Then calculate his maximized utility level, or the indirect utility function, u* (px ,py , m).
(d) (2 points) Assume the lowest acceptable level of utility is U(¯) . Set up Mark’s expenditure minimization problem.
(e) (9 points) Solve Mark’s expenditure minimization problem for his Hicksian/Com-pensated demand functions xc (px , pg ,U(¯)) and yc (px , pg ,U(¯)). Then calculate his minimized expenditure level, or the expenditure function, E(px , pg ,U(¯)). (Hint:
You don’t need to verify the conditions again, and you can just use the tangency condition from (c) if the Lagrangian method is appropriate for (c).)
(f) (6 points) State the definitions of Shephard’s Lemma for both good x and y. Then, verify that for good y. Note that if Shephard’s Lemma doesn’t hold, then you must made an algebra mistake in the previous part.
(g) (3 points) Under what condition(s) the Marshallian/Ordinary demand function is equivalent to the Hicksian/Compensated demand function, i.e. x* = xc. (Hint: There should be two mutually exclusive conditions. Just state the conditions cor- rectly. No algebra or proof required.)