Hello, if you have any need, please feel free to consult us, this is my wechat: wx91due
Phys 110A - Electromagnetism
Homework 7
Spring 2024
Due date: Friday May 31 at 11:59 pm. Upload on Gradescope. Late work with 20% penalty will be accepted no later than Saturday June 1 at 11:59 pm.
Guidelines: Refer to the document titled ‘Homework Instruction and Grading’ available on the Gau-chospace website. The problem numbers are taken from Griffiths, 4th Edition. If you are using a different edition of the textbook, please make sure that you solve the right problems.
Problems:
1. Griffiths 5.4 (It’s the same problem in the third, fourth, and fifth editions.)
2. Griffiths 5.7 (It’s the same problem in the third, fourth, and fifth editions.)
Add the following parts to the question:
(a) Solve the problem using an alternative method (i.e., not using the hint provided) by viewing the current as composed of a collection of moving point charges.
(b) Consider a thick wire with a uniform volume current density J (like in example 5.4 of Griffiths). If you try to directly apply the result of this problem to a section of the wire, the result seems to contradict the steady-state nature of the configuration. How do you resolve this? Answer it from the perspective of both the ways of solving this problem (i.e., the approach using the hint in the textbook, as well as that of part (a) above).
3. Problem 5.10 (It’s the same problem in the third, fourth, and fifth editions.)
4. Problem 5.46 (Problem 5.44 in the third edition, and 5.49 in the fifth edition.)
5. The main goal of this problem is to find the torque that acts on a planar current loop in a uniform externally generated magnetic field. The uniform field B points in some direction in space. The shape and size of the (planar) loop are arbitrary; we may think of the current as being supplied by twisted leads on which any net force will be zero.
(a) We can orient our coordinates so that B is perpendicular to the ˆx axis, and our current loop lies in the x − y plane, as shown in Figure 1. Argue that it is always possible to choose such a coordinate system.
(b) Consider some small element of the loop, and work out its contribution to the torque about the ˆx axis. Only the ˆz component of the force on it will be involved, and hence only the ˆy component of the field B, which we have indicated as ˆyBy in the diagram. Set up the integral that will give the total torque. Show that this integral will give, except for constant factors, the area of the loop.
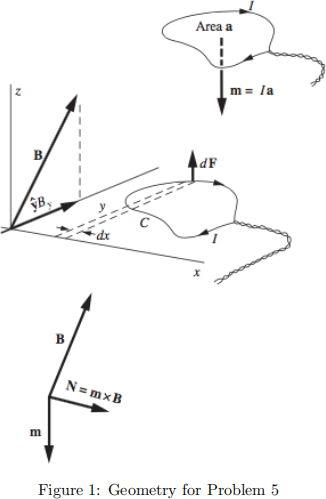
(c) The magnetic moment of a current loop is defined as a vector m whose magnitude is Ia, where I is the current and a is the area of the loop, and whose direction is normal to the loop with a right-hand-thread relation to the current, as shown in Figure 1. Show now that your result implies that the torque N on any current loop is given by the vector equation
N = m × B (0.1)
(d) What is the net force on the loop?