Homework 2
STATS 4A03
Due on Crowdmark by Friday, February 9th at 11:59pm
Guidelines: Unless otherwise specified, you are required to justify and prove all your answers.
You are welcome and encouraged to collaborate with other students on homework as-signments, and you should feel free to discuss the problems and talk about how to come up with solutions with each other. However, you are expected to write all your solutions independently of any collaborators and you should not share written solutions with other students before the deadline. If you collaborate with other students, you must cite any collaborators that you had on any given problem.
You may use the textbook and lecture slides. You are discouraged from using outside resources (online, Math stack, etc.), but if you decide to do so, you must cite all your sources. If your solution is too similar to the cited one, you may lose credit on the problem.
Your homework grade will be based on completeness plus the correctness of a random subset of four (4) problems.
Exercise 1. Suppose {Yt} is an AR(1) process with |φ| < 1. Find the autocovariance func-tion of Wt = ∇Yt = Yt − Yt−1 in terms of φ and σe 2 (the variance of white noise). Then show that Var(Wt) = 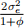 .
.
Exercise 2. Consider the ARMA(1, 2) model Yt = 0.8Yt−1 + et + 0.7et−1 + 0.6et−2. Show that ρk = 0.8ρk−1 for k > 2 and ρ2 = 0.8ρ1 + 
Exercise 3. Consider two MA(2) processes: one with θ1 = θ2 = 1/6 and another with θ1 = −1, θ2 = 6. Show that both processes have the same autocorrelation function. Then find the roots of the characteristic polynomial of both processes. How do they compare?
Exercise 4. Let {Yt} be a stationary process with ρk = 0 for k > 1. Prove that |ρ1| ≤ 1/2. (Hint: Consider Var(Yn+1 + Yn + · · · Y1) and Var(Yn+1 − Yn + Yn−1 − · · · + Y1), and use the fact that both variances must be nonnegative for all n.)
Exercise 5. Let {et} be a zero-mean, unit-variance white noise process. Consider a process {Yt} which starts at time t = 0, and is defined recursively as follows: Let Y0 = c1e0, Y1 = c2Y0 + e1, and Yt = φ1Yt−1 + φ2Yt−2 + et for t ≥ 2 (as in an AR(2) process).
a) Show that the process has zero mean.
b) For values of φ1, φ2 in the stationarity region for an AR(2) process, show how to choose c1, c2 such that both Var(Y0) = Var(Y1) and the lag 1 autocorrelation cov(Y0, Y1) equals that of a stationary AR(2) process with parameters φ1, φ2.
c) Once the process {Yt} has been generated, show how one can transform in into a new process which has any desired mean and variance. (Remark. This gives a method for simu-lating stationary AR(2) processes.)
Exercise 6. Consider the ARMA(1, 1) model Yt = 0.3Yt−1 − 0.7et−1 + et . Prove that {Yt} is invertible and write out its invertible representation.