Hello, if you have any need, please feel free to consult us, this is my wechat: wx91due
ECON60662: Tutorial 2
Q1. An infinitely lived agent maximizes his lifetime utility with consumption c in each time period. Her elasticity of marginal utility of consumption is θ, and the rate of time preference isp. The government imposes a proportional income tax τ, and a proportional consumption tax σ, and provides a lump-sum subsidy ϕ . The firms produce output with a production function y = AKα , where y is output per capita and k is capital per capita.
(a) Write the optimisation problem for the agent in her lifetime.
(b) Solve the above problem to obtain the growth rate of consumption. Explain the conditions necessary to generate long run growth.
(c) What is the effect on the growth rate of an increase in either of the following variables: τ, σ, and ϕ . Explain the results.
(d) If the subsidy ϕ is proportional on output, how will it change the results above?
Q2. In an overlapping generations economy, a two-period-lived agent born at time tis endowed with 1 unit of time, and does the followings in her lifetime:
I. at her young age, t:
i. She spends lt(t) amount of time on work for output production with the state of technology At : yt(t) = Atlt(t) (2.1)
ii. She spends the rest of her time for gaining leisure.
iii. She gains utility from consuming ct(t) , enjoying leisure.
II. at her old age, t+1:
i. She spends lt(t)+1 amount of time on work for output production with the state of technology
At+1:
yt(t)+1 = At+1lt(t)+1 (2.2)
ii. She spends the rest of her time for gaining leisure.
iii. She gains utility from consuming ct(t)+1, enjoying leisure.
Note that β ∈ (0, 1) is the weight on future consumption and leisure compared to their current ones, andy > 0 is the weight on leisure over consumption in the same period.
(a) Write the amount of time she allocates for leisure at her young and old age. (b) Write the optimisation problem for the agent in her lifetime.
(c) Solve the above problem to find the optimal labour supplies in the first and second periods of agent’slife.
(d) Suppose that technology evolves according to At+1 = (1 + Lt )At, where Lt is total labour supply at time t. Assuming that the population of agents is N, derive an expression for the growth of technology.
(e) Derive an expression for growth of output per capita.
Q3. The decision problem for an infinitely-lived agent is given as follows:
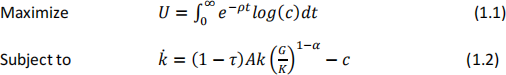
where ( P, A> 0, α ∈(0,1) )c denotes consumption, k denotes capital, τ denotes a (constant) proportional income tax, G denotes government expenditures, and Y denotes output. The total population of agents is fixed and normalised to one.
(a) Explain the functions.
(b) Solve the above problem to obtain the agent’s Euler equation. Does it confirm long run growth?
(c) State the government’s budget constraint and use this to obtain an expression for the growth rate of consumption as a function of τ .
(d) Derive the optimum τ and explain why the government can’t impose τ beyond this value.
(e) Explain the conditions necessary to generate long run growth in this decision making problem.
Q4. Consider an economy in which monopolistically competitive firms produce differentiated intermediate goods that are used in the production of final output. The decision problem for a firm each period is given as follows:
maximise π = px一Yx (5.1)
subject to x = p一α (5.2)
(Y > 0, α >1) where π denotes profits, x denotes output and p denotes the price of output. An intermediate input is created through research and development at a fixed cost of k . The total
(discounted) payoff from innovation is

where r is the (constant) rate of interest.
i. Solve the firm’s profit maximisation problem to determine the optimal price for its product. What is the implication for the time path of profits?
ii. Using the free-entry condition (V = 0 ), derive an expression for r in terms of π and k . If the
Euler equation for households is c![]() c = r 一P, where c denotes consumption of final output, what is the effect on equilibrium consumption growth of an increase in the fixed cost of innovation?
c = r 一P, where c denotes consumption of final output, what is the effect on equilibrium consumption growth of an increase in the fixed cost of innovation?