Hello, if you have any need, please feel free to consult us, this is my wechat: wx91due
Econ 23950, Midterm, Fall 2023
Part I. TRUE-FALSE Questions (39 points)
Please answer the following questions as TRUE or FALSE, and provide a justification using at most 4-5 sentences for each of the questions. Where larger points are given, you should describe your answer more fully. The questions should be answered in the context of the theory and mate-rials discussed in class or done in homework. A good answer often involves identifying particular theoretical concepts related to the question. Points earned depend entirely on clarity of your argu-ments. No points will be given for an answer without a justification or restatement of the given statement.
(8 pts) 1. When the government takes away resources from you based on proportionate taxation on your economic activity and give them back to you in a lump-sum transfer, distortion disappears.
False. When the tax is rebated back in a lump-sum transfer, the income effect associated with the taxation is neutralized. The substitution effect, which causes distortions, would still be present. Students need to explain the nature/definition of distortions.
(8 pts) 2. When the social welfare function takes the Rawlsian form, consumption expenditures should be equalized across the rich and poor households.
The SWF takes a form of max{min{ϕ1u1, ϕ2u2, ϕ3u3, ...}}. This implies that level sets for SWF are described as L-shaped indifference curves if we have two arguments (u1, u2). This further implies that the maximium of the SWF occurs at a kink point, which implies that, as long as we set the weights equal to each other, u1 = u2. This does not mean, however, that the arguments in the utility functions are the same. We could have different utility functions for different individuals and we also have labor supply affecting the utility function. For example, we could have productive people having higher labor supply and high consumption and unproductive people lower labor supply and lower consumption, as long as their utility levels are the same.
(8 pts) 3. A proportional tax on wealth, whether financial or physical, can be levied as a non-distortionary taxation. For full credit, justify your answers fully with carefully specifying conditions.
This tax will cause no distortion under two conditions. First, the tax comes as a surprise so that people (and businesses) cannot change the amount of wealth on which the tax is levied. Second, the tax will not be invoked in the future and people (and businesses) believe that there will be no future wealth tax. Otherwise, they will change their savings behavior and adjust their wealth levels to avoid taxes.
Quite a few students simply described the Ramsey taxation results we derived in class without addressing the time inconsistency issue or inelastic supply of k0. They tend to describe that τt = τt+1 and τt c = −τt w are the conditions (which is incorrect). I gave only 3 points for an answer that looks like memory-dumping.
(15pts) 4. Read the following excerpt from an article published on July 12, 2023 on the website of the Illinois Answers Project and write an essay:
“Brandon Johnson (current Mayor of Chicago) promised during the campaign to raise $800 million in new revenue, all without touching property taxes, which are typically how cities come up with the money they need in a pinch.
But with ideas like a financial transactions tax and a business head tax already hitting road-blocks in Springfield and the City Council, some supporters say an income tax on high earners may be one of the mayor’s only remaining options for raising significant revenues.
Johnson has not endorsed a city income tax. But a plan backed by many of his allies and supporters would levy a 3.5% tax on all household income above $100,000. Supporters say it could generate more than ✩2 billion in new revenue for Chicago, all without hitting its poorest residents or touching property taxes. ”
Prompt for your essay: If you work as a chief budget officer for the City of Chicago, how do you go about estimating the additional revenue from such income tax? To structure your essay, first consider the following method:
1. For each tax return, you find income above ✩100k and multiply that by 3.5%.
2. You then sum these taxes across all the households in the city.
Discuss why this is a bad method, based on the concepts/methodology we developed in this course. Secondly, propose a method (perhaps a model) that allows you to better estimate the revenue. Note that you do not need to write equations. Remember that I am trying to assess your mastery of this course through your essay–your reasoning and arguments should be grounded on your learning in this course.
For full credit, they need to clarify that the current behaviors are a poor guide to future behaviors when the rules of the game change (Lucas Critique). For a better method to estimate the tax revenue, they can approach either theoretically or empirically. Theoretically, they need to provide a detailed description of the model that allows them to calculate people’s incomes and corresponding tax revenues as a function of the income tax rate. They can mimic the methodology we developed in Model II (capital but no labor with proportional tax) in deriving the expression for the tax revenue as a function of the tax rate. Empirically, they could estimate labor supply elasticities for different segments of the labor force to estimate how the new tax affect their labor supply decisions and income generating activities.
Almost all of them got the point that the tax bases will change in response to the newly introduced tax. Some students took issue with the tax itself and discussed why the new tax itself was a bad idea. If their arguments are grounded by reasoning discussed in class, I gave them some credit. Quite a few students described Laffer curve as a “method” to estimate the tax revenue. They need to describe how they estimate the Laffer curve itself, either theoretically or empirically.
Part II. Does Lower Corporate Income Taxation Affect Wages for Workers? (54 points)
Read the questions carefully. If you cannot show your math, show the steps you would follow, write what the equations should look like, and explain your results intuitively. Below, I have written the questions in a way that, even if you cannot do a question, you can still solve subsequent questions.
You often hear people criticize the “trickle-down” economics. Namely, they argue that tax cuts for high-income households and corporations won’t help working families. This question allows you to think about that through the lens of a general equilibrium modeling.
Suppose that the representative household maximizes the following lifetime utility:
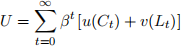
subject to the following budget constraint:
Ct + bt+1 + It + Tt = πt + (1 − τt k )RtKt + (1 − τt w)wtLt + (1 + rt)bt
along with the evolution of capital:
Kt+1 = (1 − δ)Kt + It
and the given initial conditions: K0 > 0, b0 = 0. We assume that taxes are levied proportionately on output so that Tt = τt kRtKt+τt wwtLt . Firms do the contmporaneous profit maximization where the profit is defined as πt ≡ f(Kt , Lt) − RtKt − wtLt The usual functional assumptions hold for u(), v(), f(). Also, assume that the government issues debt Bt+1 every period to meet the following period-by-period budget constraint where {Gt}∞t=0 is predetermined and fixed:
Gt + (1 + rt)Bt = Tt + Bt+1
(6 pts) 1. Set up the Lagrangian for the representative household and derive the first order conditions.
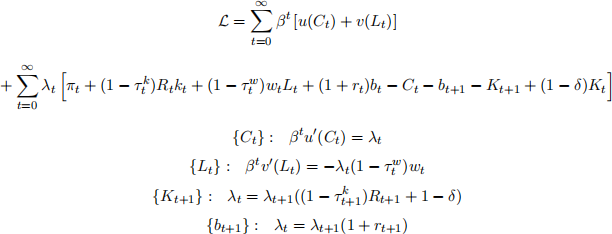
(6 pts) 2. Derive the optimality conditions for the household, both intertemporal and intratemporal. Explain intuitively.
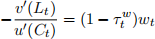
The MRS between labor and consumption should be set equal to the after tax wage (relative price of labor in units of consumption).
u ′ (ct) = βu′ (ct+1)((1 − τt k +1)Rt+1 + 1 − δ)
u ′ (ct) = βu′ (ct+1)(1 + rt+1)
The intertemporal marginal rate of subsitution should be set equal to the rate of return on saving (bonds or physical capital)
(6 pts) 3. Describe the firm’s profit maximization problem and derive the optimality conditions. Explain.
max f(Kt , Lt) − wtLt − RtKt
Kt,Lt
FOCs:
{Lt} : fL(Kt , Lt) = wt
{Kt} : fK(Kt , Lt) = Rt
Marginal product should be set equal to the factor price.
(8 pts) 4. Discuss the market clearing conditions and derive the following equations (if you cannot derive them, explain why these equations must hold):
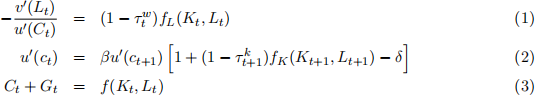
There are four markets and three prices in this economy (the price of goods is the numeraire). When the labor market clears, the household’s MRS equation (labor supply schedule) and the firm’s FOC (labor demand schedule) must intersect, leading to equation (1). When the capital market clears, the household’s EE equation (capital supply schedule) and the firms’ FOC (capital demand schedule) must intersect, leading to equation (2). When the credit market clears, the government’s debt Bt+1 must equal the household’s saving bt+1 for all time periods. Finally, the goods market clearing condition says that the supply of goods has to equal the aggregate demand for goods, which leads to equation (3).
There is actually a typo in Equation (3). It should be
Ct + Gt + Kt+1 − (1 − δ)Kt = f(Kt , Lt)
If the student identified this error, please give them extra credit. The solutions to the subsequent questions should not change, though.
In the subsequent questions, we will explore the question of “Does a lower corporate tax increase wages?”. For that, we will focus our analysis on steady state where Kt+1 = Kt = K; Ct+1 = Ct = C; Lt+1 = Lt = L; τt k +1 = τt k = τ k ; τt w +1 = τt w = τ w; Gt+1 = Gt = G. For simplicity, continue to treat G as exogenously given. You do not have think about the link between τ k and τ w.
(8 pts) 5. Describe the general method you will implement to answer the question. You may want to start by rewriting the above equations (1)-(3) at steady state as (1)’, (2)’, and (3)’. Note that you do not have to do any algebra. Define the object you want to solve and describe the steps to characterize the object. Hint: implicit differentiation.
Dropping the time-subscripts all together and making the solutions as functions of τk, we can rewrite (1)-(3) as
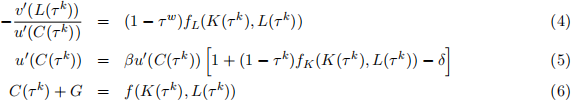
We can differentiate implicitly all three equations with respect to τ k . This results in 3 equations for 3 unknowns: { ∂τ ∂Kk , ∂τ ∂Lk , ∂τ ∂Ck }. We can then solve and characterize the object of our interest: ∂τ ∂Lk .
Again, Equation (6) ought to be
C(τ k ) + G + δK = f(K(τ k ), L(τ k ))
but ignore the discrepancy. The procedure should be the same.
(6 pts) 6. Now forget about the general method. Suppose the production function takes a Cobb-Douglas formulation: f(Kt , Lt) = Kt αL 1−αt. Using the Euler equation evaluated at the steady state, derive the expression for k ≡ L/K at steady state as a function of τ k (and β, δ, α). Hint: you should have seen a similar expression before in class.
We can rewrite equation (5) as
1 = β [1 + (1 − τ k )αKα−1L 1−α − δ] = β [1 + (1 − τ k )αkα−1 − δ]
Solving this with respect to k yields
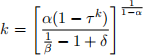
(6 pts) 7. Continue to assume the Cobb-Douglas production function. Derive the expression for the labor demand schedule as a function of τ k . Is w an increasing/decreasing function in τ k ? Explain. Hint: Use the firm’s FOC for profit maximization and use the result obtained in the previous question.
w = fL(K, L) = (1 − α)KαL −α = (1 − α)k α
Using the solution from the previous question,
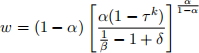
w is a decreasing function in τ k . This is because an increase in capital income tax reduces capital accumulation at steady state, which reduces marginal product of labor for workers. This causes a leftward (inward) shift in the labor demand schedule.
(8 pts) 8. Based on your result above, does a lower capital income tax create more jobs? Why or why not? Explain intuitively.
If the labor supply schedule remains fixed, an increase in the labor demand schedule will result in higher w and higher L. However, the labor supply schedule has C in its argument. Hence, we will need to specify v() and u() functions to see exactly how C and L depend on τ k . If they say that τ w will have to rise when τ k increases (as long as Gs are fixed), that there will be no income effect, that they will increase labor supply, then give them full credit.