STAT512 Homework 8

STAT512

Homework 8

a. Identify the design

b. Construct a profile plot to display the level of interaction between grape cultivar and weed control.

c. Perform appropriate F tests, and draw conclusions from these tests concerning the effect of grape cultivar and weed control on the mean growth.

d. Perform multiple comparisons using Tukey’s procedure if necessary.

e. Use a normal probability of residuals and plot of residuals vs. predicted values to determine if there are violations in the appropriate conditions for validly drawing conclusions from the F tests.

2. An agricultural experiment station is investigating the appropriate planting density for three commercial varieties of tomatoes: celebrity, sunbeam, and trust. The researcher decides to examine the effects of four planting densities: 5, 20, 35, and 50 thousand plants per hectare. The experiment station has three large fields that would be appropriate for the study. At each of the fields, 12 plots are prepared, and the 12 treatments are randomly assigned to the plots. A separate randomization is done at each of the three fields.

Celebrity                  Sunbeam Trust

Density                  Density                       Density

a. Identify the design.

b. Construct a profile plot to display the level of interaction between treatment and level.

c. Perform appropriate F tests, and draw conclusions from these tests concerning the effect of variety and planting density on the mean yield of the tomato plants.

d. Perform multiple comparisons using Tukey’s procedure if necessary.

e. Use a normal probability of residuals and plot of residuals vs. predicted values to determine if there are violations in the appropriate conditions for validly drawing conclusions from the F tests.

3. Wheat cultivation practices such as seeding rate, row spacing, and date of planting have direct effects on the crop yield. Cultivation practices to optimize production are established with experiments on newly introduced wheat cultivars.

Research Objective: In one such instance, a researcher wanted to determine the optimal seeding rate for newly introduced durum wheat with a high semolina extract important in the making of pasta.

Treatment design: Five seeding rates (30, 80, 130, 180, and 230 lb/acre) were used for the treatment design. Based on other cultivars common to the area, these seeding rates should have bracketed the rate for optimal production.

Experimental Design: The experiment was conducted in an irrigated field with the water gradient along one direction of the experimental area. In addition, the experimental fields on this farm were known to have soil gradients created by the grading required to make the land suitable for irrigation. These soil differences were generally perpendicular to the irrigation runs.

The researcher blocked the fields plots in a row and column arrangement to control for soil and water gradients in two directions on the experimental field. The seeding rate treatments were randomized to the field plots in 5 X 5 Latin square arrangement.

The layout of the experimental plots in a Latin square design after randomization is shown in the Display below. The grain yield in each plot in hundredweight (100lb) per acre is shown in each plot along with the treatment letter.

The field row blocks coincide with the irrigation gradient, and the column row blocks coincide with the soil gradient perpendicular to the irrigation gradients. The treatments are arrayed in a Latin square arrangement with each of the treatments appearing once in each row block and once in each column block.

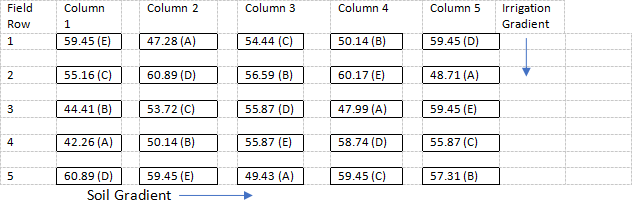
Source: Dr. M. Ottman, Department of Plant Sciences, University of Arizona

a. Identify the design.

b. Perform appropriate F test, and draw conclusions from the test concerning the effect of seeding rate on the mean yield.

c. Perform multiple comparisons using Tukey’s procedure if necessary.

d. Use a normal probability of residuals and plot of residuals vs. predicted values to determine if there are violations in the appropriate conditions for validly drawing conclusions from the F test.